

With this fact in mind, we can draw EC and create triangle ECD. If a triangle is inscribed in a parallelogram (or a rectangle) such that a side of the triangle coincides with a side of the parallelogram (or the rectangle) and a vertex not on of this side of the triangle is on the opposite side of the parallelogram (or the rectangle), then the area of the triangle is exactly half of the area of the parallelogram (or the rectangle). To solve this problem, we need to know the following fact: (2) Line AH, the altitude of parallelogram ABCD, is 5. What is the area of parallelogram ABCD (figure not drawn to scale)? Untitled.pngIn the picture, quadrilateral ABCD is a parallelogram and quadrilateral DEFG is a rectangle. I know that Magoosh has a few hundred math questions, all appropriate difficulty for the GMAT, and each followed but its own video solution. I am not as familiar with Kaplan questions overall, I am not qualified to make a statement about them. You don't have to have be Isaac Newton to answer the hardest questions.
The GMAT doesn't expect that, even on 800 level questions.
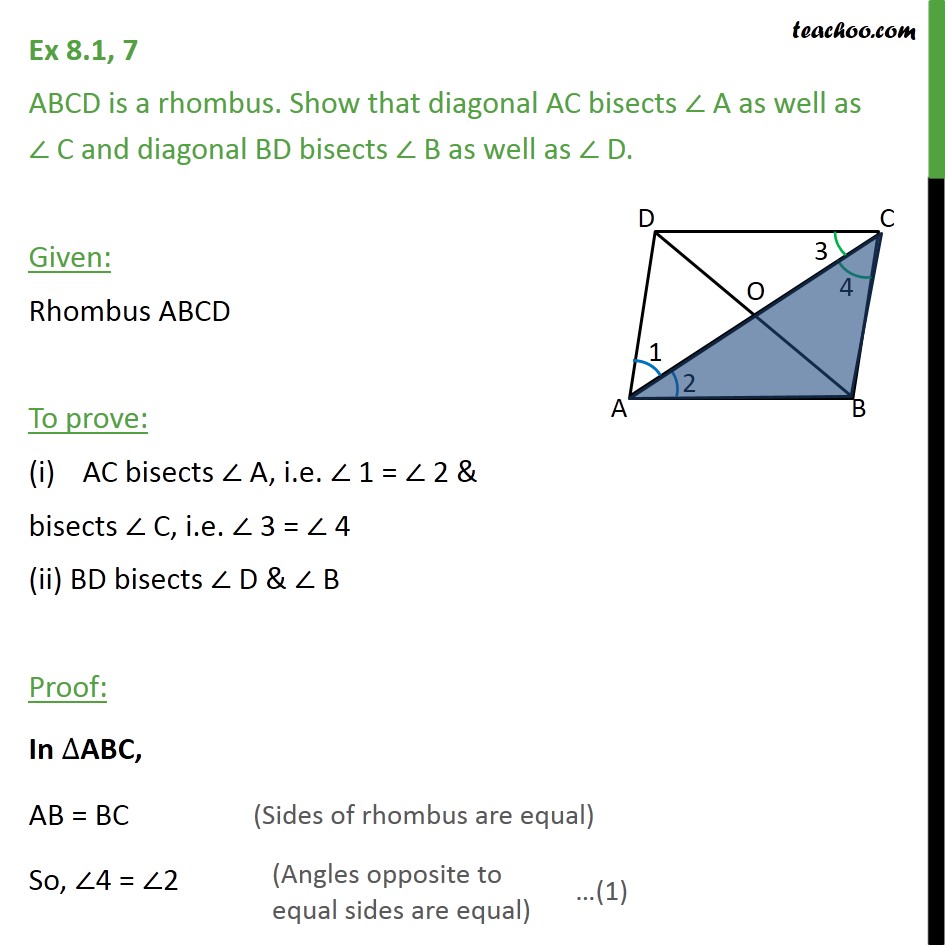
#Parallelogram abcd full
If you've never seen this theorem, there's virtually no way that you will derive the full geometry proof in under a minute, unless you operate at Isaac Newton level. Any GMAT math question, no matter how challenging, is something that someone facile with math would be able to solve in under a minute. I would say a question like this - a question that hinges on a relatively obscure geometry theorem that one probably would have to prove from scratch to answer the question - is something far harder than what they would put on the GMAT. Whereas the questions on GMATPREP seem to be much simpler than this, No? The more I see Kaplan questions, the more I feel the questions can be extremely hard. What is the likelihood of such a question on the GMAT. You just need to substitute side for side.ĭear Mike. I must admit I couldn't get this right, but after reading the explanation of mikemcgarry, I think this way is simpler as you don't have to think and prove similars. So (3) can be rewritten as EQ * DC = EF * DE. But in rectangle DEFG, CP = EF (since DEFG is rectangle, CP perpendicular with DE, so CP must = EF) Now, for triangle DEC, consider ED as base and CP as height, we have Area of DEC = 1/2 CP * DE (2)įrom (1) and (2), the 2 area is the same, we have EQ * DC = CP * DE (3).
Now, from C, draw a line CP that is perpendicular with DE with P is on DE.
#Parallelogram abcd how to
The tricky part is how to link it with the rectangle DEFG. It also equals 1/2 Area ABCD (area of parallelogram is base * height).

I use the same diagram that mikemcgarry provided.įirst, we all agree that by considering DC as base and EQ as height, Area DEC = 1/2 * EQ * DC (1). I think it's doesn't need to be that complicated. Hi, mikemcgarry's is good but it uses similar triangles to prove. Please let me know if you have any questions on what I've said here. Here's another geometry DS, a little closer to the actual level of difficulty of the GMAT itself. Therefore, Statement #2 is insufficient.ĭoes all this (including everything in the pdf) make sense? If we know the altitude and not the base, that's not enough. Statement #2: Line AH, the altitude of parallelogram ABCD, is 5.Īrea of a parallelogram = (base)*(altitude). Leaving those details aside for the moment, Statement #1 is sufficient.
#Parallelogram abcd pdf
Why do the rectangle and parallelogram have equal area? You will see the complete geometric argument in the pdf attachment to this post. Well, to cut to the chase, this statement is sufficient because the rectangle and the parallelogram must have equal area. Statement #1: The area of rectangle DEFG is 8√5. As a geometry geek myself, I found this a very cool geometry problem, but I will say - it is WAY harder than anything you would be expected to figure out for yourself on the real GMAT.


 0 kommentar(er)
0 kommentar(er)
